2010 Finalists | Best Illusion of the Year Contest

Impossible motion: magnet-like slopes

2010 First prize

Kokichi Sugihara

Meiji Institute for Advanced Study of Mathematical Sciences, Japan

In this video, wooden balls roll up the slopes just as if they are pulled by a magnet. The behavior of the balls seems impossible, because it is against the gravity. The video is not a computer graphic, but a real scene. What is actually happening is that the orientations of the slopes are perceived oppositely, and hence the descending motion is misinterpreted as ascending motion. This illusion is remarkable in that it is generated by a three-dimensional solid object and physical motion, instead of a two-dimensional picture.

Counter-intuitive illusory contours

2010 Second prize

Bart Anderson

University of Sydney, Australia

In many natural scenes, portions of occluding surfaces and contours can be camouflaged by having an identical color as their background. It is now well known that the visual system will often generate illusory contours and surfaces in order to fill-in this missing information. It is widely believed that the visual system does this in order to provide the best overall “explanation” of the images that form in our eyes. In this illusion, four circular disks are simply translated back and fourth over a thin square outline. Although all of the image data is fully “explained” by the visible disks, the visual system generates a strong and clearly visible additional illusory contour inside the square outline. This illusion cannot be understood with any known mechanism or theory that has been proposed to explain the formation of illusory contours. This quicktime movie is best viewed by downloading it and playing it in “loop” mode.

Two sinusoids: 6 – 1 perceptions

2010 Third prize

Jan Kremlacek

Charles University in Prague, Czech Republic

This movie requires Flash Player 9

Multiplication of stationary and moving sinusoid builds a moving curve with five perceptible and one hardly traceable interpretation. After a short observation a jerky leftwards motion (1st percept) transforms to a rigid rotating double-helix (2nd) or a flexible weaving ribbon (3rd). Both structures derived from the motion are ambiguous and can be perceived as rotating or waving in one of two ways (4th and 5th). Displaying the curve as dots bouncing up and down without any change of their horizontal position creates 6th percept, which is difficult to follow. To explore all multistable perceptions use the movie cues.

Stretching out in the tub

Lydia Maniatis

American University, USA

This illusion is based on a billboard showing a bathtub shot at an angle. As we walk from one end of the picture to the other, the bathtub seems to stretch and shrink. Why? Each change in location results in a different retinal image. When processed in the usual way, each of these images results in a different 3D percept. Walking past the real bathtub will also produce a series of retinal images, different from those produced by the picture. All of these images will elicit a single, common 3D interpretation, and thus shape constancy.

The steerable spiral

Peter Meilstrup & Michael Shadlen

University of Washington, USA

We show an array of moving spots. Each spot travels in a spiral counterclockwise towards the center of the screen, but contains a grating that moves opposite to its direction of travel. When each spot is by itself its direction of travel is clear. But when we arrange the spots so they are closer together on the screen, they appear to move in different directions — either inward or outward, and either clockwise or counterclockwise, depending on how we arrange them. The proximity of each spot to its neighbors “steers” the perceived direction of motion.

The monkey-business illusion

Daniel Simons

University of Illinois, USA

You might have heard about my earlier demonstration in which people fail to notice a person in a gorilla suit when they are busy counting how many times a group of people pass a basketball (see www.theinvisiblegorilla.com). People can only experience the gorilla effect once-after they know to look for a gorilla, it is no longer unexpected. But what would happen if we tried showing you another video just like it? If you know that something unexpected might happen, and you actively look for unexpected events, does that make you more likely to notice them? Try it for yourself.

Daniel Simons’s presentation of “The Monkey Business Illusion” at the Best Illusion of the Year contest in 2010. He gave the presentation while wearing a gorilla suit.

The blurry heart illusion

Kohske Takahashi, Ryosuke Niimi & Katsumi Watanabe

University of Tokyo, Japan

Please move the eyes over the static red hearts on the blue background. The blurry heart wobbles whereas the sharp heart remains still.

The fat face thin (fft) illusion

Peter Thompson

University of York, UK

It is well-known that faces are more difficult to recognise when they’re upside-down and that sometimes we misperceived the facial expressions of upside-down faces as is shown in ‘The Margaret Thatcher illusion’.
Now I give you ‘The Fat Face Thin illusion’. Compare the upside-down face on the left of the lower figure with the upright face on the right. The upside-down version looks much thinner, – altogether a longer shaped face than the upright version. Of course the pictures are identical. This illusion illustrates the internal features of the face, (eyes, nose, mouth) can distort our perception of face shape.

Attention-biased after-image rivalry

Peter Tse

Dartmouth College, USA

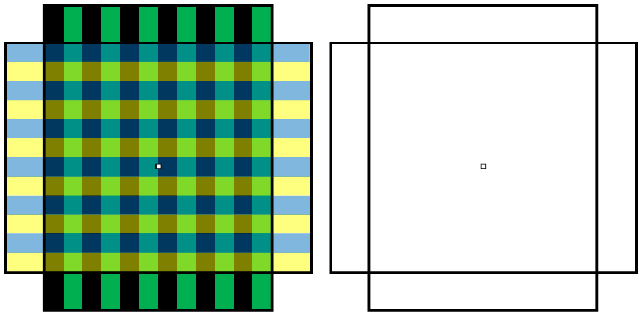
Fixate the colored image by looking at the fixation spot for about 60 seconds. Now shift your eyes to the fixation spot surrounded by rectangular outlines. If you attend to the vertical outline rectangle you will see the afterimage corresponding to it, and if you attend to the horizontal outline rectangle you will see the different afterimage corresponding to it. You can shift which afterimage you experience by attending to one rectangle and then the other.

Illusory gloss

Maarten Wijntjes & Sylvia Pont

Delft University of Technology, The Netherlands

The movie shows a Brownian surface (which has a fractal structure, but this does not seem to be important for the illusion). The appearance seems to change between matte and glossy, as the ruler illustrates. However, the surface is continuously rendered with matte (Lambertian) reflectance. What is happening is that the height of the surface is stretched in the viewing direction. The illumination direction is transformed together with the stretching to keep the cast shadows constant. This combined transformation of the light and surface height results in an illusory gloss. We initially though that the effect could be attributed to a luminance histogram skew transformation, but this seems not the case. A simple version of the current explanation is that stretching a surface together with the light direction (the so-called Bas-Relief transformation), induces illusory highlights due to darkening at points where the surface normal points away from the optical axis.

Search